Help! My Peaks Look Strange - Fronting and Tailing in GC

Overloading Effects in Gas Chromatography

Norbert Reuter, Technical Manager, Global Technical Support for CSD, Middelburg, The Netherlands

Introduction

Overloading effects are strongly related to the sample capacity of stationary phases in gas chromatography. If this sample capacity is exceeded, the peak shapes are influenced and therefore give an indication of the effect. Overloading always shows in fronting peaks and influences the resolution within a chromatogram. For accurate analyses (robust quantification, etc.) overloading should be avoided.

Wall-Coated Open Tubular Columns (WCOT)

In WCOT columns, we have liquid stationary phases and partitioning chromatography. Partitioning means one or more components are distributed between the mobile phase (carrier gas) and the stationary phase. The process can be described as a mixture of the components in the phases.

Henry’s Law (Equation 1) describes thermodynamically mixtures of gases in liquids. It says: “At a constant temperature, the amount of a given gas dissolved in a given type and volume of liquid is directly proportional to the partial pressure of that gas in equilibrium with that liquid.”

Henry's Law:                p_i = k_H · c_i                                                                                                                                                                                                        (1)
p = partial pressure of the solute in the gas phase
k_H = Henry constant
c = concentration of the solute in the liquid phase

For dilute mixtures, the relation is linear and is the basis of the ideal Gaussian peaks shapes. WCOT columns have high phase ratios, meaning that the stationary phase is the minority phase.

Phase ratio for capillary columns:              β = V_gas / V_liquid = 250 · df / ID                                                                                                     (2)
V_gas = Volume of the gas phase
V_liquid = Volume of the liquid phase
df = film thickness in [µm]
ID = Internal diameter in [mm]

Overloading occurs when the sample capacity of the liquid phase is exceeded; Henry’s Law is no longer valid and deviates from linearity. There is more sample present in the carrier gas and this sample is transported with the carrier gas flow to the end of the column and arrives there earlier than expected. The peak shows fronting.

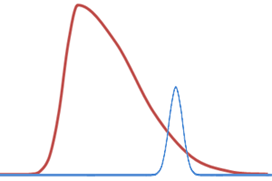
               (a) WCOT column overloading                                             (b) PLOT column overloading

Figure 1: Peak shapes of an overloaded peak (dark red) vs. ideal peaks (light blue)

Porous-Layer Open Tubular Columns (PLOT)

Henry’s Law is only valid for mixtures of a gas in a liquid and, therefore, for partitioning chromatography. PLOT columns are used for adsorption chromatography; here Henry’s Law has to be exchanged with the Langmuir adsorption equation (Equation 3).

Langmuir equation:          θ = α · P_i / (1 + α · P_i)                                                                                                                            (3)
θ = fractional surface coverage
α = Adsorption constant
P = partial gas pressure or concentration

      
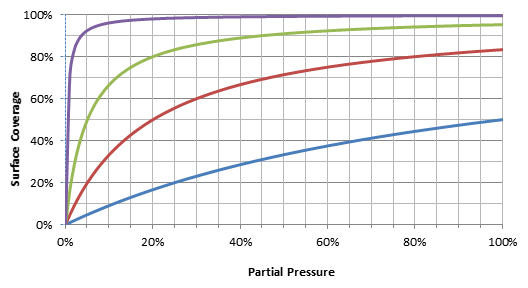          Figure 2: Langmuir adsorption isotherms for different adsorption constants

With increasing partial pressure, the surface cannot adsorb sample components any longer. The active places are already occupied, and the overloading happens then in the gas phase, producing often a triangular peak shape. The peak starts earlier than expected and the peak ends at the normal position. Due to the shape it seems that the peak shows tailing, which is not the case (see Figure 1b). The best region for separations on PLOT columns is in the linear range of the Langmuir isotherm. In the example here: up to 100% in the partial pressure range for the blue, 20% for red, 5% for green and 2% for the violet line.

Sample Capacity

The sample capacity is for every combination of sample component and liquid or solid phase different. For the liquid phases, imagine the solution of salt and sugar in a glass of water. The maximum solubility for salt is ca. 380 g/L, whereas for sugar it is ca. 675 g/L.

To make it even more complicated, the maximum salt (sugar) solubility in water at 20 °C is 380 g/L (675 g/L), but 400 g/L (810 g/L) at 90 °C. The solubility is temperature-dependent and changes along the temperature program in a gas chromatographic run! For gases, there is also a pressure-dependence, because in equilibrium the gaseous component would rather dissolve in the phase than being in the carrier gas.

But there is also a positive point; the sample capacity is independent from the number of different components. In the salt/sugar example, the water would dissolve 380 g salt and 675 g sugar in 1 L at the same time.

In the empirical and pragmatic theory of mass capacity, the maximum amount of component can be estimated by Equation 4:

w_max   =   a · V_phase · (1 + 1/k) / √ N_th   =   a · 2π · ID · df · (1 + 1/k) / √ N_th                              (4)
w_max = maximum amount of component
a = characteristic constant of the phase/component interaction
V_phase = Volume of the liquid stationary phase
k = retention factor of component
N_th = plate number
ID = internal diameter of the column 
df = film thickness

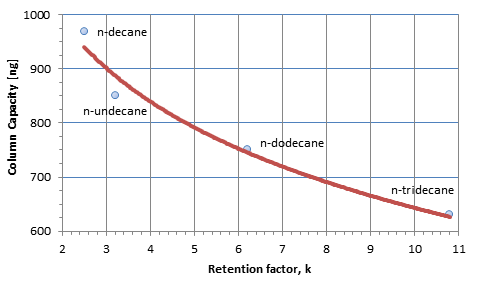
The characteristic constant, a, can be determined by experiments. The sample capacity is also dependent on the size of the component or rather its retention factor. The bigger the retention factor, the smaller is the sample capacity. In the picture of size, bigger molecules occupy more space in the phase and, therefore, you need fewer molecules to saturate the phase (see Figure 2).

                  Figure 3: Sample capacity of a 25 m x 0.53 mm x 2.0 µm CP-Sil 5 CB column at 100 °C

Also, in all these cases the chromatographic likes-like principle is valid. Apolar components dissolve in an apolar phase easily and show almost no overloading. A polar component like acetic acid, for example, will dissolve less easily in an apolar phase and is therefore quickly overloaded. For polar components, a polar phase would be better.

Overloading in its extreme form is called flooding and flooding effects distort peak shapes until they are almost unidentifiable. They lead to peak shapes that almost look like Christmas trees and the effect is also called the Christmas-tree effect. It is related to too much injected volume and miniature temperature variations in the mix zone, giving much peak splitting.

Overloading and Resolution

Overloading leads to broader peaks with broader peak widths; if you remember the resolution equation, broader peaks decrease the resolution.

Resolution:     R_s   =   2 · Δ(t_R) / Σ(b_0.5)    =    2 · (t_R,2 - t_R,1) / (b_0.5,1 + b_0.5,2)                                            (5)
t_R = retention times of peaks 1 and 2
b_0.5 = peak width at half height of peaks 1 and 2

Purnell equation:          R_s   =   ¼ · (α - 1) · k_av / (k_av + 1) · √ N_th                                                                                         (6)

α = selectivity 
k_av = average retention factor for peaks 1 and 2
N_th = plate number

Plate number:          N_th   =    5.54 · (t_R / b_0.5)²                                                                                                                                                      (7)

t_R = retention time
b_0.5 = peak width at half height

If the denominator increases, the resolution decreases (Equation 5). If the retention factors and the phase selectivity do not change during overloading, the resolution is proportional to the square root of the plate number (Equation 6). The plate number is inversely proportional to the square of the peak width at half height (Equation 7). If the peak width increases by 10%, the resolution decreases by 10%. The user must decide, what the acceptable decrease in resolution is, depending on the application.

Solutions

There is a solution to all overloading effects: reduce sample amounts or increase the amount of phase.

1. The amount of phase can be increased using thicker films, longer columns (more phase, but also more diffusion and band broadening) and/or using columns with bigger internal diameters. The injection volume needs to be constant
2. The reduction in sample amount can be achieved by injecting less, using a higher split flow
3. A third solution is working at higher temperatures (better dissolution of components in the phase)

Estimated Sample Capacities of Capillary Columns

            Table 1: Estimated sample capacities for different column dimensions

Conclusions

To avoid overloading in gas chromatography, you can:

• Inject less
• Use higher split flows (more goes to vent, less onto the column)
• Use columns with thicker films (more film, more loadability)
• Use columns with bigger internal diameters (also more film and more loadability)
• Work at elevated temperatures (thermodynamic influence, solubility in phase increases)

• A combination of all these items