Hello, i'm a chemistry student and i'm trying to do a method validation.
I'm trying to calculate the LOD and the LOQ of my method, but i realize there're multiple ways to do so.
In the ICH HARMONISED GUIDELIN (2022 R2) they suggest to use this statistic approach for the LOQ and le LOD:
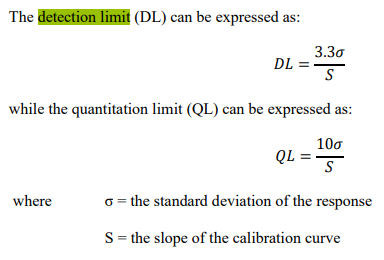
Now may i ask you if you have any paper/book where this statistic method is demonstrated? I've done plenty of research, but no one goes deep enough to explain how to obtain the "3.3" and the "10" factors. I would like to know the whole mathematical demonstration so i can argue judge better what i am using for the validation.
Sorry for disturbing you
and thank you even if you can't answer!
